Celestine,-_ElvinaAmelia_QiannanYe_ArtificialAutomation_Part2

3.1  BEAUTY IN MATHEMATICS

Architecture is hugely influenced by the perspective of beauty in math. [9]

3.1.1 Golden ratio

"Phi" (Golden Ratio or Golden Section): ={1.618}{033}{988}{7} \ Φ=1.6180339887…

The golden ratio has been applied to various works by artists and architects since ancient

times. For example The Parthenon, Notre Dame, The Taj Mahal.

Figure 9

3.1.2 Centers

The research conducted by Koman is that when space is divided into multiple parts, each part will have a new center. When these parts form irregular subdivisions, it is possible that the center of each small part is not the same as the original center position. The brand new centers will create completely different patterns.

Figure 10

3.1.3 Boundaries

Boundaries are related to geometry. Two points can connect to form a line, plus the third point can be connected into a triangle. The triangle can translate into many kinds of polygons. The final format is close to the circle.

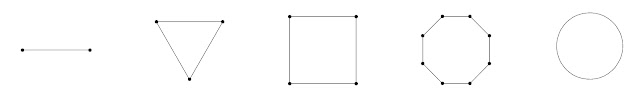Figure 11

3.1.4 Symmetries

When an item has its own symmetrical axis, it can easily complete the whole form.  One of the outcomes of the mathematical beauty is the mirror effect.

Figure 12

3.1.5 Deviation

A slight change in the value can cause deviations. The error can bring diversity to the whole, and also vary in density, structure, quantity, and strength.

Figure 13

However, there have been studies on the fundamental of beauty from the point of view of our brain. Neuroaesthetic is an attempt at solving the very complex question of the definition of beauty.[10] It is possible that AI can assist us to finally come to a definitive conclusion if AI develops beauty by instinct.

3.2 MATHEMATICAL APPLICATION IN ARCHITECTURE DESIGN

There have been many approaches to applying mathematical devices by contemporary architects. As computation technology thrives in the last few decades, architectural design has been able to broaden and innovate its formal and/or organizational variations. Here are some existing examples on design outcome generated by mathematical algorithms.

3.2.1 Tessellation

Gerbis suggested that the tessellations are defined by the coding of mathematics where shapes are arranged in repetition without gaps or overlapping each other. There are a few forms of tessellation, they consist of the tessellation of regular geometric shapes, fractal tessellation and Voronoi tessellation.[11]

Figure 14: Celling of Hazrate-Masomeh’s mosque in Qom, Iran

3.2.2 Fractal

Fractal is a never-ending pattern, the similar pattern repeats itself across different scales to form a complex pattern. This can be observed in Figure 15. Fractals occur a lot in the nature such as trees, leaves, shells, rivers and snowflakes.

Figure 15: Example of a 3D fractal pattern

3.2.3 Mathematical geometries and curves

Throughout his career, Antoni Gaudi had been designing architecture using pure geometrical forms and mathematical curves, albeit using manual tools. For example, his most historic project, Sagrada Familia, was designed using a prototype made of hanging chains. This method was utilized to simulate the physics in order to find the formal language that will be detrimental to its construction. [12]

Figure 16: (Left) Sagrada Familia chain model. (Right) Sagrada Familia ceiliing

The advancement of this formal language is popularized by the late Zaha Hadid. She used spline geometry in parametric design as can be seen in figure 17.

Figure 17: King Abdullah Financial District Metro Station by Zaha Hadid Architects

3.2.4 Generative algorithmic agency

Generative design methodology using an algorithmic agency such as swarm and material physics.

Figure 18: AADRL Swarm Printing: aerial robotic bridge construction

3.2.5 Symmetrical ornaments in high-resolution

The combination of complex computation with the sense of familiarity found in symmetry.

Figure 19: Digital Grotesque I by Michael Hansmeyer and Benjamin Dillenburger